Curve Surveying
INTRODUCTION :
During the review of the arrangement of a task including street or railroads, the course of the line may Change because of some unavoidable conditions.
The point of the adjustment in course is known as the avoidance edge. For it to be feasible for a vehicle to run effectively along the street or railroad track, the two straight lines are associated by a circular segment which is known as the bend of the street or track.
At the point when the bend is given in the flat plane , it is known as a level bend.
The point of the adjustment in course is known as the avoidance edge. For it to be feasible for a vehicle to run effectively along the street or railroad track, the two straight lines are associated by a circular segment which is known as the bend of the street or track.
At the point when the bend is given in the flat plane , it is known as a level bend.
Definition &Types Of Curve Surveying :
Definition of Curves:
Twists are standard turns given in the lines of correspondence like boulevards, railways, etc and moreover in conduits to accomplish the consistent modify obviously. They are also used in the vertical plane at all movements of assessment to avoid the unexpected distinction in assessment at the apex.
Curves gave in the level plane to have the consistent adjust in course are known as Horizontal twists, while those gave in the vertical plane to get the dynamic change in assessment are known as vertical twists. Twists are spread out on the ground along within line of the work. They may be indirect or logical.
Curves gave in the level plane to have the consistent adjust in course are known as Horizontal twists, while those gave in the vertical plane to get the dynamic change in assessment are known as vertical twists. Twists are spread out on the ground along within line of the work. They may be indirect or logical.
Types Of Curve
(i) Simple,
(ii) Compound
(iii) Reverse and
(iv) Deviation
(i) Simple Curve :
A fundamental twist involves a lone round portion of a circle interfacing two straights. It has length of a comparative degree all through. In fig. 11.1 T1 D T2 is the essential curve with T1O as its range. |
| Simple Curve |
(ii) Compound Curve:
A compound twist contains in any event two essential curves having different radii bending a comparative way and lying on a comparative side of the customary deviation. Their spotlights lie on a comparable side of the twist. In fig. 11.2, T1 P T2 is the compound twist with T1O1 and PO2 as its radii.
 |
| Compound Curve |
(iii) Reverse Curve:
A pivot or serpentine twist is included two bends having proportionate or unmistakable radii contorting in opposite manners with a commonplace diversion at their convergence. Their centers lie of backwards sides of the twist. In fig. 11.3 T1 P T2 is the pivot twist with T1O1 and PO2 as its radii.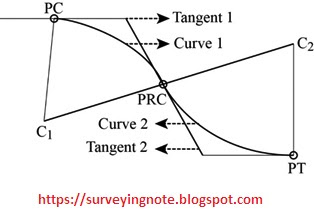 |
| Reverse Curve |
Rearrange twists are used when the straights bend equal or cross at a little edge. They are typically used in railroad sidings and now and again on rail line tracks and boulevards suggested for low speeds. They should be kept up a vital good ways from past what many would consider conceivable on rule railroad lines and streets where velocities are essentially high.
(iv) Deviation Curve:
A deviation twist is fundamentally a mix of two switch twists. It is used when it ends up imperative to wander from a given straight route in order to swear off intervening obstacles, for instance, a bend of conduit, a structure, etc. In fig. 11.4. T1 EDFT2 is the deviation twist with T1O, EO2 and FO2 as its radii.
 |
| Deviation Curve |
Names of Various Parts of a Curve:
(I) The two straight lines AB and BC, which are related by the curve are known as the deviations or straights to the twist.
(ii) The motivations behind combination of the two straights (B) is known as the intersection point or the vertex.
(iii) When the twist sidetracks to the right half of the headway of study as in fig. 11.5, it is named as right given curve and when aside, it is named as left given twist.
(iv) The lines AB and BC are deviations to the curves. Stomach muscle is known as the chief deviation or the back diversion BC is known as the subsequent straying or the forward diversion.
(v) The centers (T1 and T2) at which the curve contacts the diversions are known as the deviation centers. The beginning of the curve (T1) is known as the deviation twist point and the completion of the twist (T2) is known as the twist diversion point.
(vi) The point between the diversion lines AB and BC (ABC) is known as the edge of intermingling (I)
(ii) The motivations behind combination of the two straights (B) is known as the intersection point or the vertex.
(iii) When the twist sidetracks to the right half of the headway of study as in fig. 11.5, it is named as right given curve and when aside, it is named as left given twist.
(iv) The lines AB and BC are deviations to the curves. Stomach muscle is known as the chief deviation or the back diversion BC is known as the subsequent straying or the forward diversion.
(v) The centers (T1 and T2) at which the curve contacts the diversions are known as the deviation centers. The beginning of the curve (T1) is known as the deviation twist point and the completion of the twist (T2) is known as the twist diversion point.
(vi) The point between the diversion lines AB and BC (ABC) is known as the edge of intermingling (I)
 |
| elemation of curve |
Components of a Simple Circular Curve
(vii) The point by which the forward deviation diverts from the back straying is known as the preoccupation edge (ɸ) of the curve.
(viii) The partition the two deviation reason for combination to the straying point is known as the diversion length (BT1 and BT2).
(ix) The line joining the two straying centers (T1 and T2) is known as the long-agreement
(x) The round section T1FT2 is known as the length of the curve.
(xi) The mid-point (F) of the roundabout section (T1FT2) in called highest point or pinnacle of the twist.
(xii) The partition from the reason for intersection highlight the apex of the twist BF is known as the pinnacle evacuate.
(xiii) The division between the apex of the curve and the midpoint of the long concordance (EF) is known as the versed sine of the twist.
(xiv) The point subtended at the point of convergence of the curve by the roundabout section T1FT2 is known as the Central edge and is equal to the shirking edge (ɸ).
(viii) The partition the two deviation reason for combination to the straying point is known as the diversion length (BT1 and BT2).
(ix) The line joining the two straying centers (T1 and T2) is known as the long-agreement
(x) The round section T1FT2 is known as the length of the curve.
(xi) The mid-point (F) of the roundabout section (T1FT2) in called highest point or pinnacle of the twist.
(xii) The partition from the reason for intersection highlight the apex of the twist BF is known as the pinnacle evacuate.
(xiii) The division between the apex of the curve and the midpoint of the long concordance (EF) is known as the versed sine of the twist.
(xiv) The point subtended at the point of convergence of the curve by the roundabout section T1FT2 is known as the Central edge and is equal to the shirking edge (ɸ).
Designation of Curves :
A twist may be doled out either by the range or by the edge subtended at within by an amicability of explicit length In India, a curve is appointed by the point (in degrees) subtended at the center by an agreement of 30 meters (100 ft.) length. This point is known as the degree of the curve.
 |
| Curve |
Let R= The radius of the curves in meters
D= The degree of the curve
MN= The chord, 30m long
P= The mid-point of the chord.
Transition Curves:
A non-round twist of fluctuating reach introduced between a straight and an indirect twist to give basic adjusts obviously of a course is known as an advancement or easement twist. It is also inserted between two pieces of a compound or switch twist.
Focal points of giving an advancement twist at each finish of a round twist:
(I) The change from the straying to the round curve and from the indirect twist to the diversion is made dynamic.
(ii) It gives satisfactory techniques for securing a persistent addition of super-ascent from zero on the diversion to the necessary full whole on the principal indirect twist.
(iii) Danger of crash, side sliding or upsetting of vehicles is cleared out.
(iv) Discomfort to explorers is slaughtered.
Focal points of giving an advancement twist at each finish of a round twist:
(I) The change from the straying to the round curve and from the indirect twist to the diversion is made dynamic.
(ii) It gives satisfactory techniques for securing a persistent addition of super-ascent from zero on the diversion to the necessary full whole on the principal indirect twist.
(iii) Danger of crash, side sliding or upsetting of vehicles is cleared out.
(iv) Discomfort to explorers is slaughtered.
Conditions to be satisfied by the change bend:
(I) It should meet the straying line similarly as the indirect curve superfluously.
(ii) The pace of addition of shape along the change twist should be equal to that of augmentation of super-tallness.
(iii) The length of the change twist should be to such a degree, that the full super-ascent is cultivated at the convergence with the round curve.
(iv) Its range at the crossing point with the indirect curve should be proportionate to that of round twist.
There are three sorts of progress twists in like way use:
(ii) The pace of addition of shape along the change twist should be equal to that of augmentation of super-tallness.
(iii) The length of the change twist should be to such a degree, that the full super-ascent is cultivated at the convergence with the round curve.
(iv) Its range at the crossing point with the indirect curve should be proportionate to that of round twist.
There are three sorts of progress twists in like way use:
(1) A cubic parabola,
(2) A cubical winding, and
(3) A lemniscate, the underlying two are used on railways and turnpikes both, while the third on streets in a manner of speaking.
Right when the advancement twists are introduced at each finish of the essential indirect twist, the mix thusly got is known as merged or Composite Curve.
(2) A cubical winding, and
(3) A lemniscate, the underlying two are used on railways and turnpikes both, while the third on streets in a manner of speaking.
Right when the advancement twists are introduced at each finish of the essential indirect twist, the mix thusly got is known as merged or Composite Curve.
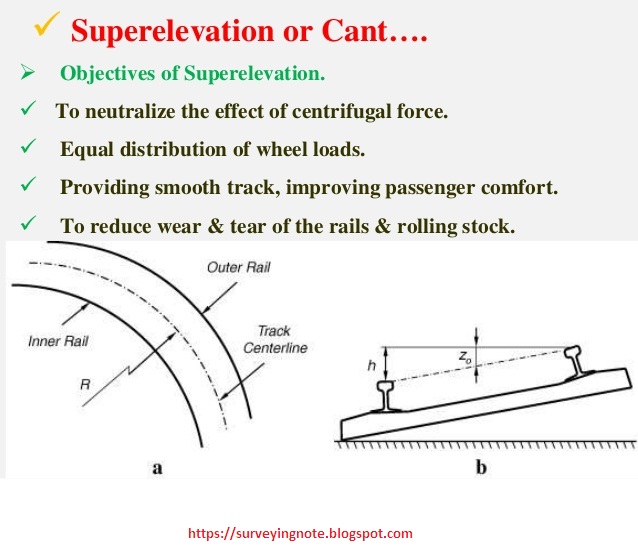
Super-Elevation or Cant:
Exactly when a vehicle goes from a directly to a twist, it is followed up on by an outward force despite its own weight, both acting through the point of convergence of gravity of the vehicle. The diffusive force follows up on a level plane and will by and large push the vehicle out of control.
In order to kill this effect the outside edge of the track is excessively raised or raised over the internal one. This raising of the outside edge over the inside one is called super rise or cant. The proportion of super-tallness depends on the speed of the vehicle and clear of the curve.
In order to kill this effect the outside edge of the track is excessively raised or raised over the internal one. This raising of the outside edge over the inside one is called super rise or cant. The proportion of super-tallness depends on the speed of the vehicle and clear of the curve.
 |
| Super elevation |
Let:
W = the greatness of vehicle acting vertically downwards.
F = the dissimilar force following up on a level plane,
v = the speed of the vehicle in meters/sec.
g = the accelerating in light of gravity, 9.81 meters/sec2.
R = the range of the twist in meters,
h = the super-stature in meters.
b = the breadth of the road or the division between the focal points of the rails in meters.
By then for congruity, the resultant of the weight and the outward force should be identical and reverse to the reaction inverse to the road or rail surface.
W = the greatness of vehicle acting vertically downwards.
F = the dissimilar force following up on a level plane,
v = the speed of the vehicle in meters/sec.
g = the accelerating in light of gravity, 9.81 meters/sec2.
R = the range of the twist in meters,
h = the super-stature in meters.
b = the breadth of the road or the division between the focal points of the rails in meters.
By then for congruity, the resultant of the weight and the outward force should be identical and reverse to the reaction inverse to the road or rail surface.
Characteristics of a Transition Curve :
Here two straights AB and BC make a redirection edge ∆, and an indirect curve EE' of clear R, with two change, twists TE and E'T' at the two terminations, has been implanted between the straights.
(I) It is evident from the expect that in order to fit in the advancement twists at the completions, around whimsical twist (T1F1T2) of imperceptibly progressively conspicuous range must be moved towards the center as(E1 EF E E1. The division through which the curve is moved is known as move (S) of the twist, and is proportional to, where L is the length of each change twist and R is the scope of the perfect round twist (EFE'). The length of the move (T1E1) and the change twist (TE) generally separate each other.
Here two straights AB and BC make a redirection edge ∆, and an indirect curve EE' of clear R, with two change, twists TE and E'T' at the two terminations, has been implanted between the straights.
(I) It is evident from the expect that in order to fit in the advancement twists at the completions, around whimsical twist (T1F1T2) of imperceptibly progressively conspicuous range must be moved towards the center as(E1 EF E E1. The division through which the curve is moved is known as move (S) of the twist, and is proportional to, where L is the length of each change twist and R is the scope of the perfect round twist (EFE'). The length of the move (T1E1) and the change twist (TE) generally separate each other.
Curve Surveying Watch :
QUESTION AND ANSWER
1 What type of curve?
= 2 type
2 Two type curve is
= horizontal and vertical
3 What type of horizontal curve?
= 5 type
4 The radius of a one-degree curve is=
= 1,719m
5 Suparelevations road formula is
= h=bV2/gR
6 Suparelevations railway formula is
= h=GV2/gR
7 Centrifugal ratio of roads
= 1/4
8 Centrifugal ratio of railway
= 1/8
9 When a curve consists of a single
arc with a constant radius connecting the two tangents, it is
= simple curve
10 When a curve consists of two or
more arcs with different radii, it is
= compound curve
11 A curve consists of two arcs
bending in opposite direction is
= reverse curve
12 A curve of variable radius is known
as a
= transition curve
13 If the angle of intersection is
= 0 = 1800-I
14 If radius not given then
= R= 1,719/D
15 Tangent length is
= R tan0/2
16 The first tangent point is also
known
= point of curve
17 The second point is also known as
= point of tangency
18 When two tangents AB and BC meet at B, the point B is known
= vertex
19 An ideal transition curve is also
known
= clothoid curve
20 An vertical curve is considered as
= parabolic curve
21 A vertical curve is designed on the
basis of
= minimum sight distance
22 In reverse curve, the
superelevation provided at the point of reverse curvature is
= zero
23 Total angle of deflection of a
transition curve is
= ¼ * spiral angle
24 The shape of a vertical curve is
= parabolic
25 The multiplying constant of a
tacheometer is
= f/I
--------------------------------------------
Chan Surveying Note -- Read
Compass Surveying Note -- Read
Plane Table Surveying Note-- Read
Levelling Survey Note -- Read
Contouring Survey Note -- Read
Theodolite Survey Note -- Read
Best Surveyor Book Buy -- Link
Thank You...













0 Comments:
Post a Comment